33
Find the vertex of the graph of
\begin{equation*}
y=-9\!\left(x-4\right)^{2}-5
\end{equation*}
34
Find the vertex of the graph of
\begin{equation*}
y=-6\!\left(x+3\right)^{2}+3
\end{equation*}
35
Find the vertex of the graph of
\begin{equation*}
y=-4\!\left(x-10\right)^{2}-9
\end{equation*}
36
Find the vertex of the graph of
\begin{equation*}
y=-2\!\left(x-3\right)^{2}+2
\end{equation*}
37
Find the vertex of the graph of
\begin{equation*}
y=0.7\!\left(x+4.1\right)^{2}+8.2
\end{equation*}
38
Find the vertex of the graph of
\begin{equation*}
y=3\!\left(x-9.1\right)^{2}-3.6
\end{equation*}
39
Write the vertex form for the quadratic function \(f\text{,}\) whose vertex is \((2,5)\) and has leading coefficient \(a=5\text{.}\)
\(\displaystyle{ f(x) =}\)
40
Write the vertex form for the quadratic function \(f\text{,}\) whose vertex is \((-5,-7)\) and has leading coefficient \(a=7\text{.}\)
\(\displaystyle{ f(x) =}\)
41
Write the vertex form for the quadratic function \(f\text{,}\) whose vertex is \((8,1)\) and has leading coefficient \(a=9\text{.}\)
\(\displaystyle{ f(x) =}\)
42
Write the vertex form for the quadratic function \(f\text{,}\) whose vertex is \((1,9)\) and has leading coefficient \(a=-8\text{.}\)
\(\displaystyle{ f(x) =}\)
43
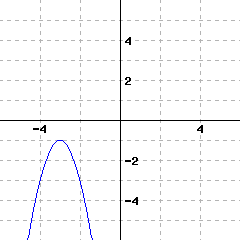
A graph of a function \(f\) is given. Use the graph to write a formula for \(f\) in vertex form. You will need to identify the vertex and also one more point on the graph to find the leading coefficient \(a\text{.}\)
\(\displaystyle{ f(x) =}\)
44
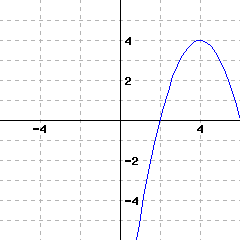
A graph of a function \(f\) is given. Use the graph to write a formula for \(f\) in vertex form. You will need to identify the vertex and also one more point on the graph to find the leading coefficient \(a\text{.}\)
\(\displaystyle{ f(x) =}\)
45
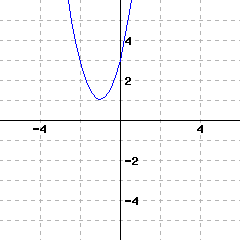
A graph of a function \(f\) is given. Use the graph to write a formula for \(f\) in vertex form. You will need to identify the vertex and also one more point on the graph to find the leading coefficient \(a\text{.}\)
\(\displaystyle{ f(x) =}\)
46
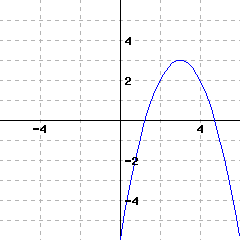
A graph of a function \(f\) is given. Use the graph to write a formula for \(f\) in vertex form. You will need to identify the vertex and also one more point on the graph to find the leading coefficient \(a\text{.}\)
\(\displaystyle{ f(x) =}\)
47
Let \(G\) be defined by \(G(x)={\left(x-6\right)^{2}-8}\text{.}\)
What is the domain of \(G\text{?}\)
What is the range of \(G\text{?}\)
48
Let \(H\) be defined by \(H(x)={\left(x+4\right)^{2}-7}\text{.}\)
What is the domain of \(H\text{?}\)
What is the range of \(H\text{?}\)
49
Let \(K\) be defined by \(K(x)={1.3\!\left(x-8\right)^{2}-9}\text{.}\)
What is the domain of \(K\text{?}\)
What is the range of \(K\text{?}\)
50
Let \(K\) be defined by \(K(x)={7.7\!\left(x+3\right)^{2}+4}\text{.}\)
What is the domain of \(K\text{?}\)
What is the range of \(K\text{?}\)
51
Let \(f\) be defined by \(f(x)={-\left(x-5\right)^{2}-1}\text{.}\)
What is the domain of \(f\text{?}\)
What is the range of \(f\text{?}\)
52
Let \(g\) be defined by \(g(x)={-8\!\left(x+6\right)^{2}-6}\text{.}\)
What is the domain of \(g\text{?}\)
What is the range of \(g\text{?}\)
53
Let \(h\) be defined by \(h(x)={7\!\left(x-\left(-1\right)\right)^{2}+\left(-1\right)}\text{.}\)
What is the domain of \(h\text{?}\)
What is the range of \(h\text{?}\)
54
Let \(h\) be defined by \(h(x)={5\!\left(x + {\frac{2}{9}}\right)^{2}+{\frac{1}{6}}}\text{.}\)
What is the domain of \(h\text{?}\)
What is the range of \(h\text{?}\)
55
Let \(F\) be defined by \(F(x)={-2\!\left(x-{\frac{1}{3}}\right)^{2} - {\frac{8}{5}}}\text{.}\)
What is the domain of \(F\text{?}\)
What is the range of \(F\text{?}\)
56
Let \(G\) be defined by \(G(x)={7\!\left(x-{\frac{8}{9}}\right)^{2}+{\frac{1}{3}}}\text{.}\)
What is the domain of \(G\text{?}\)
What is the range of \(G\text{?}\)
57
Consider the graph of the equation \(y={\left(x-5\right)^{2}-3}\text{.}\)
Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units
and units .58
Consider the graph of the equation \(y={\left(x-7\right)^{2}+9}\text{.}\)
Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units
and units .59
Consider the graph of the equation \(y={\left(x-93.7\right)^{2}+29.6}\text{.}\)
Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units
and units .60
Consider the graph of the equation \(y={\left(x+82.5\right)^{2}-39.3}\text{.}\)
Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units
and units .61
Consider the graph of the equation \(y={\left(x+\frac{2}{3}\right)^{2}+\frac{8}{3}}\text{.}\)
Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units
and units .62
Consider the graph of the equation \(y={\left(x+\frac{8}{7}\right)^{2}+\frac{7}{4}}\text{.}\)
Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units
and units .