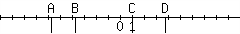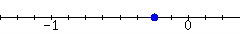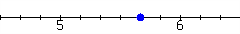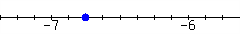ORCCA Fractions and Fraction Arithmetic

Section A.2 Fractions and Fraction Arithmetic

The word “fraction” comes from the Latin word fractio, which means “break into pieces.” For thousands of years, cultures from all over the world have used fractions to understand parts of a whole.

Figure A.2.0.1. Alternative Video Lesson

Subsection A.2.1 Visualizing Fractions

Parts of a Whole.

One approach to understanding fractions is to think of them as parts of a whole.

In Figure A.2.1.2, we see $1$ whole divided into $7$ parts. Since $3$ parts are shaded, we have an illustration of the fraction $\frac{3}{7}\text{.}$ The denominator $7$ tells us how many parts to cut up the whole; since we have $7$ parts, they're called “sevenths.” The numerator $3$ tells us how many sevenths to consider.

a rectangle that is seven times as wide as it is tall; the entire rectangle is shaded; there is a 1 in the center of the rectangle; to its right, it is labeled 'one whole'; another rectangle of the same size is aligned directly below the first rectangle; it is subdivided equally into seven squares; the first three squares are shaded; there is a 1/7 in the center of each of the first three squares; to its right, it is labeled 'three sevenths' — Figure A.2.1.2. Representing $\frac{3}{7}$ as parts of a whole.

Checkpoint A.2.1.3. A Fraction as Parts of a Whole.

We can also locate fractions on number lines. When ticks are equally spread apart, as in Figure A.2.1.4, each tick represents a fraction.

eps pdf png svg tex

Figure A.2.1.4. Representing $\frac{3}{7}$ on a number line.

Checkpoint A.2.1.5. A Fraction on a Number Line.

Division.

Fractions can also be understood through division.

For example, we can view the fraction $\frac{3}{7}$ as $3$ divided into $7$ equal parts, as in Figure A.2.1.6. Just one of those parts represents $\frac{3}{7}\text{.}$

eps pdf png svg tex

Figure A.2.1.6. Representing $\frac{3}{7}$ on a number line.

Checkpoint A.2.1.7. Seeing a Fraction as Division Arithmetic.

Subsection A.2.2 Equivalent Fractions

It's common to have two fractions that represent the same amount. Consider $\frac{2}{5}$ and $\frac{6}{15}$ represented in various ways in Figures A.2.2.8–A.2.2.10.

one rectangle that is five times as wide as it is tall; the entire rectangle is shaded; there is a 1 in the center of the rectangle; a second rectangle that is also five times as wide as it is tall; it is subdivided equally into five squares; the first two squares are shaded; there is a 1/5 in the center of each of the first two squares; a third rectangle that is also five times as wide as it is tall; it is subdivided equally into fifteen adjacent rectangles; the first six of these smaller rectangles are shaded; there is a 1/15 in the center of each of the first six smaller rectangles — Figure A.2.2.8. $\frac{2}{5}$ and $\frac{6}{15}$ as equal parts of a whole

eps pdf png svg tex

Figure A.2.2.9. $\frac{2}{5}$ and $\frac{6}{15}$ as equal on a number line

eps pdf png svg tex

Figure A.2.2.10. $\frac{2}{5}$ and $\frac{6}{15}$ as equal results from division

Those two fractions, $\frac{2}{5}$ and $\frac{6}{15}$ are equal, as those figures demonstrate. In addition, both fractions are equal to $0.4$ as a decimal. If we must work with this number, the fraction that uses smaller numbers, $\frac{2}{5}\text{,}$ is preferable. Working with smaller numbers decreases the likelihood of making a human arithmetic error and it also increases the chances that you might make useful observations about the nature of that number.

So if you are handed a fraction like $\frac{6}{15}\text{,}$ it is important to try to reduce it to “lowest terms.” The most important skill you can have to help you do this is to know the multiplication table well. If you know it well, you know that $6=2\cdot3$ and $15=3\cdot5\text{,}$ so you can break down the numerator and denominator that way. Both the numerator and denominator are divisible by $3\text{,}$ so they can be “factored out” and then as factors, cancel out.

\begin{align*} \frac{6}{15}\amp=\frac{2\cdot3}{3\cdot5}\\ \amp=\frac{2\cdot\cancelhighlight{3}}{\cancelhighlight{3}\cdot5}\\ \amp=\frac{2\cdot1}{1\cdot5}\\ \amp=\frac{2}{5} \end{align*}

Checkpoint A.2.2.11.

Sometimes it is useful to do the opposite of reducing a fraction, and build up the fraction to use larger numbers.

Checkpoint A.2.2.12.

Subsection A.2.3 Multiplying with Fractions

Example A.2.3.13.

Suppose a recipe calls for $\frac{2}{3}$ cup of milk, but we'd like to quadruple the recipe (make it four times as big). We'll need four times as much milk, and one way to measure this out is to fill a measuring cup to $\frac{2}{3}$ full, four times:

four measuring cups, each filled two-thirds with liquid

eps pdf png svg tex

When you count up the shaded thirds, there are eight of them. So multiplying $\frac{2}{3}$ by the whole number $4\text{,}$ the result is $\frac{8}{3}\text{.}$ Mathematically:

\begin{align*} 4\cdot\frac{2}{3}\amp=\frac{4\cdot2}{3}\\ \amp=\frac{8}{3} \end{align*}

Fact A.2.3.14. Multiplying a Fraction and a Whole Number.

When you multiply a whole number by a fraction, you may just multiply the whole number by the numerator and leave the denominator alone. In other words, as long as $d$ is not $0\text{,}$ then a whole number and a fraction multiply this way:

\begin{equation*} a \cdot \frac{c}{d} = \frac{a\cdot c}{d} \end{equation*}

Example A.2.3.15.

We could also use multiplication to decrease amounts. Suppose we needed to cut the recipe down to just one fifth. Instead of four of the $\frac{2}{3}$ cup milk, we need one fifth of the $\frac{2}{3}$ cup milk. So instead of multiplying by $4\text{,}$ we multiply by $\frac{1}{5}\text{.}$ But how much is $\frac{1}{5}$ of $\frac{2}{3}$ cup?

If we cut the measuring cup into five equal vertical strips along with the three equal horizontal strips, then in total there are $3\cdot5=15$ subdivisions of the cup. Two of those sections represent $\frac{1}{5}$ of the $\frac{2}{3}$ cup.

a measuring cup divided evenly into three horizontal sections, but also evenly into five vertical sections; in total, there are fifteen sections; the two that correspond to the lower two-thirds and leftmost one-fifth are shaded

eps pdf png svg tex

In the end, we have $\frac{2}{15}$ of a cup. The denominator $15$ came from multiplying $5$ and $3\text{,}$ the denominators of the fractions we had to multiply. The numerator $2$ came from multiplying $1$ and $2\text{,}$ the numerators of the fractions we had to multiply.

\begin{align*} \frac{1}{5}\cdot\frac{2}{3}\amp=\frac{1\cdot2}{5\cdot3}\\ \amp=\frac{2}{15} \end{align*}

Fact A.2.3.16. Multiplication with Fractions.

As long as $b$ and $d$ are not $0\text{,}$ then fractions multiply this way:

\begin{equation*} \frac{a}{b} \cdot \frac{c}{d} = \frac{a\cdot c}{b \cdot d} \end{equation*}

Checkpoint A.2.3.17.

Subsection A.2.4 Division with Fractions

How does division with fractions work? Are we able to compute/simplify each of these examples?

$3\div\frac{2}{7}$
$\frac{18}{19}\div5$
$\frac{14}{3}\div\frac{8}{9}$
$\frac{\ \frac{2}{5}\ }{\frac{5}{2}}$

We know that when we divide something by $2\text{,}$ this is the same as multiplying it by $\frac{1}{2}\text{.}$ Conversely, dividing a number or expression by $\frac{1}{2}$ is the same as multiplying by $\frac{2}{1}\text{,}$ or just $2\text{.}$ The more general property is that when we divide a number or expression by $\frac{a}{b}\text{,}$ this is equivalent to multiplying by the reciprocal $\frac{b}{a}\text{.}$

Fact A.2.4.18. Division with Fractions.

As long as $b\text{,}$ $c$ and $d$ are not $0\text{,}$ then division with fractions works this way:

\begin{equation*} \frac{a}{b} \div \frac{c}{d} = \frac{a}{b}\cdot\frac{d}{c} \end{equation*}

Example A.2.4.19.

With our examples from the beginning of this subsection:

$\begin{aligned}[t] 3\div\frac{2}{7}\amp=3\multiplyright{\frac{7}{2}}\\ \amp=\divideunder{3}{1}\cdot\frac{7}{2}\\ \amp=\frac{21}{2}\\ \ \end{aligned}$
$\begin{aligned}[t] \frac{18}{19}\div5\amp=\frac{18}{19}\div\divideunder{5}{1}\\ \amp=\frac{18}{19}\multiplyright{\frac{1}{5}}\\ \amp=\frac{18}{95}\\ \ \end{aligned}$
$\begin{aligned}[t] \frac{14}{3}\div\frac{8}{9}\amp=\frac{14}{3}\multiplyright{\frac{9}{8}}\\ \amp=\frac{14}{\highlight{1}}\cdot\frac{\highlight{3}}{8}\\ \amp=\frac{\highlight{7}}{1}\cdot\frac{3}{\highlight{4}}\\ \amp=\frac{21}{4} \end{aligned}$
$\begin{aligned}[t] \frac{\ \frac{2}{5}\ }{\frac{5}{2}}\amp=\frac{2}{5}\highlight{{}\div{}}\frac{5}{2}\\ \amp=\frac{2}{5}\multiplyright{\frac{2}{5}}\\ \amp=\frac{4}{25}\\ \ \end{aligned}$

Checkpoint A.2.4.20.

Subsection A.2.5 Adding and Subtracting Fractions

With whole numbers and integers, operations of addition and subtraction are relatively straightforward. The situation is almost as straightforward with fractions if the two fractions have the same denominator. Consider

\begin{equation*} \frac{7}{2} + \frac{3}{2} = 7\text{ halves}+3\text{ halves} \end{equation*}

In the same way that $7$ tacos and $3$ tacos make $10$ tacos, we have:

\begin{equation*} \begin{array}{ccccc} 7\text{ halves}{}\amp+{}\amp 3\text{ halves}\amp=\amp 10\text{ halves} \\ \frac{7}{2}{}\amp+{}\amp\frac{3}{2}\amp=\amp\frac{10}{2} \\ \amp \amp \amp=\amp5 \\ \end{array} \end{equation*}

Fact A.2.5.21. Adding/Subtracting with Fractions Having the Same Denominator.

To add or subtract two fractions having the same denominator, keep that denominator, and add or subtract the numerators.

\begin{align*} \frac{a}{b}+\frac{c}{b}\amp=\frac{a+c}{b}\amp\frac{a}{b}-\frac{c}{b}\amp=\frac{a-c}{b} \end{align*}

If it's possible, useful, or required of you, simplify the result by reducing to lowest terms.

Checkpoint A.2.5.22.

Whenever we'd like to combine fractional amounts that don't represent the same number of parts of a whole (that is, when the denominators are different), finding sums and differences is more complicated.

Example A.2.5.23. Quarters and Dimes.

Find the sum $\frac{3}{4}+\frac{2}{10}\text{.}$ Does this seem intimidating? Consider this:

$\frac{1}{4}$ of a dollar is a quarter, and so $\frac{3}{4}$ of a dollar is $75$ cents.
$\frac{1}{10}$ of a dollar is a dime, and so $\frac{2}{10}$ of a dollar is $20$ cents.

So if you know what to look for, the expression $\frac{3}{4}+\frac{2}{10}$ is like adding $75$ cents and $20$ cents, which gives you $95$ cents. As a fraction of one dollar, that is $\frac{95}{100}\text{.}$ So we can report

\begin{equation*} \frac{3}{4}+\frac{2}{10}=\frac{95}{100}\text{.} \end{equation*}

(Although we should probably reduce that last fraction to $\frac{19}{20}\text{.}$)

This example was not something you can apply to other fraction addition situations, because the denominators here worked especially well with money amounts. But there is something we can learn here. The fraction $\frac{3}{4}$ was equivalent to $\frac{75}{100}\text{,}$ and the other fraction $\frac{2}{10}$ was equivalent to $\frac{20}{100}\text{.}$ These equivalent fractions have the same denominator and are therefore “easy” to add. What we saw happen was:

\begin{align*} \frac{3}{4}+\frac{2}{10}\amp=\frac{75}{100}+\frac{20}{100}\\ \amp=\frac{95}{100} \end{align*}

This realization gives us a strategy for adding (or subtracting) fractions.

Fact A.2.5.24. Adding/Subtracting Fractions with Different Denominators.

To add (or subtract) generic fractions together, use their denominators to find a common denominator. This means some whole number that is a whole multiple of both of the original denominators. Then rewrite the two fractions as equivalent fractions that use this common denominator. Write the result keeping that denominator and adding (or subtracting) the numerators. Reduce the fraction if that is useful or required.

Example A.2.5.25.

Let's add $\frac{2}{3}+\frac{2}{5}\text{.}$ The denominators are $3$ and $5\text{,}$ so the number $15$ would be a good common denominator.

\begin{align*} \frac{2}{3}+\frac{2}{5}\amp=\frac{2\multiplyright{5}}{3\multiplyright{5}}+\frac{2\multiplyright{3}}{5\multiplyright{3}}\\ \amp=\frac{10}{15}+\frac{6}{15}\\ \amp=\frac{16}{15} \end{align*}

Checkpoint A.2.5.26.

Subsection A.2.6 Mixed Numbers and Improper Fractions

A simple recipe for bread contains only a few ingredients:

$1\,\sfrac{1}{2}$	tablespoons yeast
$1\,\sfrac{1}{2}$	tablespoons kosher salt
$6\,\sfrac{1}{2}$	cups unbleached, all-purpose flour (more for dusting)

Each ingredient is listed as a mixed number that quickly communicates how many whole amounts and how many parts are needed. It's useful for quickly communicating a practical amount of something you are cooking with, measuring on a ruler, purchasing at the grocery store, etc. But it causes trouble in an algebra class. The number $1\,\sfrac{1}{2}$ means “one and one half.” So really,

\begin{equation*} 1\,\frac{1}{2}=1+\frac{1}{2} \end{equation*}

The trouble is that with $1\,\sfrac{1}{2}\text{,}$ you have two numbers written right next to each other. Normally with two math expressions written right next to each other, they should be multiplied, not added. But with a mixed number, they should be added.

Fortunately we just reviewed how to add fractions. If we need to do any arithmetic with a mixed number like $1\,\sfrac{1}{2}\text{,}$ we can treat it as $1+\frac{1}{2}$ and simplify to get a “nice” fraction instead: $\frac{3}{2}\text{.}$ A fraction like $\frac{3}{2}$ is called an improper fraction because it's actually larger than $1\text{.}$ And a “proper” fraction would be something small that is only part of a whole instead of more than a whole.

\begin{align*} 1\,\frac{1}{2}\amp=1+\frac{1}{2}\\ \amp=\frac{1}{1}+\frac{1}{2}\\ \amp=\frac{2}{2}+\frac{1}{2}\\ \amp=\frac{3}{2} \end{align*}

Exercises A.2.7 Exercises

Review and Warmup

1.

Which letter is -13/4 on the number line?

eps pdf png svg tex

2.

Which letter is 15/4 on the number line?

eps pdf png svg tex

3.

The dot in the graph can be represented by what fraction?

eps pdf png svg tex

4.

The dot in the graph can be represented by what fraction?

eps pdf png svg tex

5.

The dot in the graph can be represented by what fraction?

eps pdf png svg tex

6.

The dot in the graph can be represented by what fraction?

eps pdf png svg tex

Reducing Fractions

7.

Reduce the fraction $\displaystyle{ \frac{7}{56} }\text{.}$

8.

Reduce the fraction $\displaystyle{ \frac{20}{45} }\text{.}$

9.

Reduce the fraction $\displaystyle{ \frac{70}{84} }\text{.}$

10.

Reduce the fraction $\displaystyle{ {{\frac{14}{15}}} }\text{.}$

11.

Reduce the fraction $\displaystyle{ \frac{168}{105} }\text{.}$

12.

Reduce the fraction $\displaystyle{ \frac{30}{6} }\text{.}$

Building Fractions

13.

Find an equivalent fraction to $\frac{3}{4}$ with denominator $20\text{.}$

14.

Find an equivalent fraction to $\frac{3}{5}$ with denominator $10\text{.}$

15.

Find an equivalent fraction to $\frac{3}{11}$ with denominator $44\text{.}$

16.

Find an equivalent fraction to $\frac{11}{13}$ with denominator $26\text{.}$

Multiplying/Dividing Fractions

17.

Multiply: $\displaystyle{ \frac{4}{9} \cdot \frac{4}{9} }$

18.

Multiply: $\displaystyle{ \frac{5}{6} \cdot \frac{5}{8} }$

19.

Multiply: $\displaystyle{ \frac{15}{7} \cdot \frac{2}{5} }$

20.

Multiply: $\displaystyle{ \frac{3}{7} \cdot \frac{8}{15} }$

21.

Multiply: $\displaystyle{8\cdot \frac{1}{3} }$

22.

Multiply: $\displaystyle{5\cdot \frac{2}{9} }$

23.

Multiply: $\displaystyle{-\frac{20}{11} \cdot \frac{7}{5}}$

24.

Multiply: $\displaystyle{-\frac{8}{7} \cdot \frac{11}{26}}$

25.

Multiply: $\displaystyle{28\cdot\left( -{\frac{6}{7}} \right)}$

26.

Multiply: $\displaystyle{10\cdot\left( -{\frac{7}{5}} \right)}$

27.

Multiply: $\displaystyle{ {{\frac{6}{49}}} \cdot {{\frac{5}{4}}} \cdot {{\frac{7}{25}}} }$

28.

Multiply: $\displaystyle{ {{\frac{5}{49}}} \cdot {{\frac{2}{25}}} \cdot {{\frac{21}{4}}} }$

29.

Multiply: $\displaystyle{ {{\frac{35}{2}}} \cdot {{\frac{1}{25}}} \cdot {6} }$

30.

Multiply: $\displaystyle{ {{\frac{21}{2}}} \cdot {{\frac{1}{49}}} \cdot {10} }$

31.

Divide: $\displaystyle{ \frac{2}{5} \div \frac{7}{4} }$

32.

Divide: $\displaystyle{ \frac{3}{8} \div \frac{5}{3} }$

33.

Divide: $\displaystyle{ \frac{1}{12} \div \left(-\frac{10}{9}\right) }$

34.

Divide: $\displaystyle{ \frac{7}{20} \div \left(-\frac{9}{25}\right) }$

35.

Divide: $\displaystyle{-\frac{16}{9} \div (-20) }$

36.

Divide: $\displaystyle{-\frac{25}{6} \div (-20) }$

37.

Divide: $\displaystyle{28 \div \frac{7}{5} }$

38.

Divide: $\displaystyle{6 \div \frac{3}{2} }$

39.

Multiply: $\displaystyle{{2 {\textstyle\frac{1}{10}}} \cdot {1 {\textstyle\frac{4}{21}}} }$

40.

Multiply: $\displaystyle{{2 {\textstyle\frac{2}{9}}} \cdot {1 {\textstyle\frac{7}{8}}} }$

Adding/Subtracting Fractions

41.

Add: $\displaystyle{\frac{3}{32} + \frac{1}{32}}$

42.

Add: $\displaystyle{\frac{17}{32} + \frac{3}{32}}$

43.

Add: $\displaystyle{\frac{4}{7} + \frac{15}{28}}$

44.

Add: $\displaystyle{\frac{4}{9} + \frac{11}{27}}$

45.

Add: $\displaystyle{\frac{4}{9} + \frac{13}{18}}$

46.

Add: $\displaystyle{\frac{1}{10} + \frac{21}{40}}$

47.

Add: $\displaystyle{\frac{2}{7} + \frac{3}{10}}$

48.

Add: $\displaystyle{\frac{1}{6} + \frac{1}{7}}$

49.

Add: $\displaystyle{\frac{1}{6} + \frac{1}{10}}$

50.

Add: $\displaystyle{\frac{3}{10} + \frac{1}{6}}$

51.

Add: $\displaystyle{\frac{5}{6} + \frac{9}{10}}$

52.

Add: $\displaystyle{\frac{4}{5} + \frac{7}{10}}$

53.

Add: $\displaystyle{-\frac{1}{11} + \frac{6}{11}}$

54.

Add: $\displaystyle{-\frac{4}{13} + \frac{12}{13}}$

55.

Add: $\displaystyle{-\frac{5}{7} + \frac{11}{21}}$

56.

Add: $\displaystyle{-\frac{4}{7} + \frac{3}{14}}$

57.

Add: $\displaystyle{-\frac{1}{6} + \frac{2}{9}}$

58.

Add: $\displaystyle{-\frac{7}{8} + \frac{6}{7}}$

59.

Add: $\displaystyle{ -1 + \frac{9}{10}}$

60.

Add: $\displaystyle{ 1 + \frac{4}{5}}$

61.

Add: $\displaystyle{ {{\frac{1}{10}}} + {{\frac{1}{6}}} + {{\frac{1}{5}}} }$

62.

Add: $\displaystyle{ {{\frac{3}{10}}} + {{\frac{2}{9}}} + {{\frac{1}{6}}} }$

63.

Add: $\displaystyle{ {{\frac{1}{5}}} + {{\frac{3}{10}}} + {{\frac{5}{6}}} }$

64.

Add: $\displaystyle{ {{\frac{7}{9}}} + {{\frac{3}{10}}} + {{\frac{1}{6}}} }$

65.

Subtract: $\displaystyle{\frac{17}{12} - \frac{7}{12}}$

66.

Subtract: $\displaystyle{\frac{29}{12} - \frac{19}{12}}$

67.

Subtract: $\displaystyle{\frac{3}{7} - \frac{11}{14}}$

68.

Subtract: $\displaystyle{\frac{5}{9} - \frac{5}{54}}$

69.

Subtract: $\displaystyle{\frac{31}{35} - \frac{2}{7}}$

70.

Subtract: $\displaystyle{\frac{44}{45} - \frac{4}{9}}$

71.

Subtract: $\displaystyle{-\frac{1}{6}-\frac{9}{10}}$

72.

Subtract: $\displaystyle{-\frac{3}{10}-\frac{5}{6}}$

73.

Subtract: $\displaystyle{-\frac{3}{10} - \left(-\frac{5}{6}\right)}$

74.

Subtract: $\displaystyle{-\frac{5}{6} - \left(-\frac{3}{10}\right)}$

75.

Subtract: $\displaystyle{ -4 - \frac{18}{5}}$

76.

Subtract: $\displaystyle{ -2 - \frac{3}{2}}$

Applications

77.

Kandace walked ${{\frac{2}{7}}}$ of a mile in the morning, and then walked ${{\frac{1}{8}}}$ of a mile in the afternoon. How far did Kandace walk altogether?

Kandace walked a total of of a mile.

78.

Tracei walked ${{\frac{1}{8}}}$ of a mile in the morning, and then walked ${{\frac{4}{11}}}$ of a mile in the afternoon. How far did Tracei walk altogether?

Tracei walked a total of of a mile.

79.

Jessica and Carl are sharing a pizza. Jessica ate ${{\frac{3}{8}}}$ of the pizza, and Carl ate ${{\frac{1}{6}}}$ of the pizza. How much of the pizza was eaten in total?

They ate of the pizza.

80.

A trail’s total length is ${{\frac{32}{63}}}$ of a mile. It has two legs. The first leg is ${{\frac{2}{7}}}$ of a mile long. How long is the second leg?

The second leg is of a mile in length.

81.

A trail’s total length is ${{\frac{14}{45}}}$ of a mile. It has two legs. The first leg is ${{\frac{1}{5}}}$ of a mile long. How long is the second leg?

The second leg is of a mile in length.

82.

James is participating in a running event. In the first hour, he completed ${{\frac{2}{9}}}$ of the total distance. After another hour, in total he had completed ${{\frac{47}{90}}}$ of the total distance.

What fraction of the total distance did James complete during the second hour?

James completed of the distance during the second hour.

83.

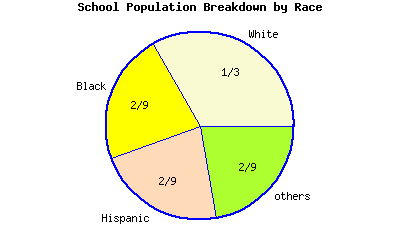
The pie chart represents a school’s student population.

eps pdf png svg tex

Together, white and black students make up of the school’s population.

84.

Each page of a book is ${6 {\textstyle\frac{3}{4}}}$ inches in height, and consists of a header (a top margin), a footer (a bottom margin), and the middle part (the body). The header is ${{\frac{5}{6}}}$ of an inch thick and the middle part is ${5 {\textstyle\frac{5}{6}}}$ inches from top to bottom.

What is the thickness of the footer?

The footer is of an inch thick.

85.

Priscilla and Adrian are sharing a pizza. Priscilla ate ${{\frac{1}{6}}}$ of the pizza, and Adrian ate ${{\frac{1}{10}}}$ of the pizza. How much more pizza did Priscilla eat than Adrian?

Priscilla ate more of the pizza than Adrian ate.

86.

Emiliano and Maria are sharing a pizza. Emiliano ate ${{\frac{2}{7}}}$ of the pizza, and Maria ate ${{\frac{1}{6}}}$ of the pizza. How much more pizza did Emiliano eat than Maria?

Emiliano ate more of the pizza than Maria ate.

87.

A school had a fund-raising event. The revenue came from three resources: ticket sales, auction sales, and donations. Ticket sales account for ${{\frac{3}{4}}}$ of the total revenue; auction sales account for ${{\frac{1}{8}}}$ of the total revenue. What fraction of the revenue came from donations?

of the revenue came from donations.

88.

A few years back, a car was purchased for ${\$23{,}500}\text{.}$ Today it is worth ${{\frac{1}{5}}}$ of its original value. What is the car’s current value?

The car’s current value is .

89.

A few years back, a car was purchased for ${\$20{,}000}\text{.}$ Today it is worth ${{\frac{1}{5}}}$ of its original value. What is the car’s current value?

The car’s current value is .

90.

The pie chart represents a school’s student population.

eps pdf png svg tex

more of the school is white students than black students.

91.

A town has $300$ residents in total, of which ${{\frac{5}{6}}}$ are Asian Americans. How many Asian Americans reside in this town?

There are Asian Americans residing in this town.

92.

A company received a grant, and decided to spend ${{\frac{1}{8}}}$ of this grant in research and development next year. Out of the money set aside for research and development, ${{\frac{4}{9}}}$ will be used to buy new equipment. What fraction of the grant will be used to buy new equipment?

of the grant will be used to buy new equipment.

93.

A food bank just received $14$ kilograms of emergency food. Each family in need is to receive ${{\frac{2}{5}}}$ kilograms of food. How many families can be served with the $14$ kilograms of food?

families can be served with the $14$ kilograms of food.

94.

A construction team maintains a ${52}$-mile-long sewage pipe. Each day, the team can cover ${{\frac{4}{5}}}$ of a mile. How many days will it take the team to complete the maintenance of the entire sewage pipe?

It will take the team days to complete maintaining the entire sewage pipe.

95.

A child is stacking up tiles. Each tile’s height is ${{\frac{2}{3}}}$ of a centimeter. How many layers of tiles are needed to reach ${12}$ centimeters in total height?

To reach the total height of ${12}$ centimeters, layers of tiles are needed.

96.

A restaurant made $300$ cups of pudding for a festival.

Customers at the festival will be served ${{\frac{1}{5}}}$ of a cup of pudding per serving. How many customers can the restaurant serve at the festival with the $300$ cups of pudding?

The restaurant can serve customers at the festival with the $300$ cups of pudding.

97.

A $2\times4$ piece of lumber in your garage is ${62 {\textstyle\frac{11}{16}}}$ inches long. A second $2\times4$ is ${55 {\textstyle\frac{7}{16}}}$ inches long. If you lay them end to end, what will the total length be?

The total length will be inches.

98.

A $2\times4$ piece of lumber in your garage is ${38 {\textstyle\frac{11}{16}}}$ inches long. A second $2\times4$ is ${44 {\textstyle\frac{7}{8}}}$ inches long. If you lay them end to end, what will the total length be?

The total length will be inches.

99.

Each page of a book consists of a header, a footer and the middle part. The header is ${{\frac{1}{9}}}$ inches in height; the footer is ${{\frac{11}{18}}}$ inches in height; and the middle part is ${3 {\textstyle\frac{4}{9}}}$ inches in height.

What is the total height of each page in this book? Use mixed number in your answer if needed.

Each page in this book is inches in height.

100.

To pave the road on Ellis Street, the crew used $4{{\frac{5}{8}}}$ tons of cement on the first day, and used $4{{\frac{9}{10}}}$ tons on the second day. How many tons of cement were used in all?

tons of cement were used in all.

101.

When driving on a high way, noticed a sign saying exit to Johnstown is $1{{\frac{3}{4}}}$ miles away, while exit to Jerrystown is $3{{\frac{1}{2}}}$ miles away. How far is Johnstown from Jerrystown?

Johnstown and Jerrystown are miles apart.

102.

A cake recipe needs ${3 {\textstyle\frac{1}{2}}}$ cups of flour. Using this recipe, to bake $9$ cakes, how many cups of flour are needed?

To bake $9$ cakes, cups of flour are needed.

Sketching Fractions

103.

Sketch a number line showing each fraction. (Be sure to carefully indicate the correct number of equal parts of the whole.)

$\dfrac{2}{3}$
$\dfrac{6}{8}$
$\dfrac{5}{4}$
$-\dfrac{4}{5}$

104.

Sketch a number line showing each fraction. (Be sure to carefully indicate the correct number of equal parts of the whole.)

$\dfrac{1}{6}$
$\dfrac{3}{9}$
$\dfrac{7}{6}$
$-\dfrac{8}{5}$

105.

Sketch a picture of the product $\frac{3}{5} \cdot \frac{1}{2}\text{,}$ using a number line or rectangles.

106.

Sketch a picture of the sum $\frac{2}{3} + \frac{1}{8}\text{,}$ using a number line or rectangles.

Challenge

107.

Given that $a\neq0\text{,}$ simplify $\displaystyle{}{\frac{3}{a}+\frac{6}{a}}\text{.}$

108.

Given that $a\neq0\text{,}$ simplify $\displaystyle{}{\frac{4}{a}+\frac{3}{2a}}\text{.}$

109.

Given that $a\neq0\text{,}$ simplify $\displaystyle{}{\frac{5}{a}-\frac{9}{5a}}\text{.}$