ORCCA More on Quadratic Functions Chapter Review

Section 12.5 More on Quadratic Functions Chapter Review

Subsection 12.5.1 Graphs and Vertex Form

In Section 12.1 we covered the use of technology in analyzing quadratic functions, the vertex form of a quadratic function and how it affects horizontal and vertical shifts of the graph of a parabola, and the factored form of a quadratic function.

Example 12.5.1 Exploring Quadratic Functions with Graphing Technology

Use technology to graph and make a table of the quadratic function \(g\) defined by \(g(x)=-x^2+5x-6\) and find each of the key points or features.

Find the vertex.
Find the vertical intercept.
Find the horizontal intercept(s).
Find \(g(-1)\text{.}\)
Solve \(g(x)=-6\) using the graph.
Solve \(g(x)\le -6\) using the graph.
State the domain and range of the function.

Explanation

The specifics of how to use any one particular technology tool vary. Whether you use an app, a physical calculator, or something else, a table and graph should look like:

\(x\)	\(g(x)\)
\(-1\)	\(-12\)
\(0\)	\(-6\)
\(1\)	\(-2\)
\(2\)	\(0\)
\(2\)	\(0\)
\(3\)	\(0\)
\(4\)	\(-2\)

the graph of the parabola g(x)=-x^2+5x-6, that opens downward, passing through the points listed in the table

eps pdf png svg tex

Additional features of your technology tool can enhance the graph to help answer these questions. You may be able to make the graph appear like:

the previous graph with all of the key points labeled as described below

eps pdf png svg tex

The vertex is \((2.5,0.25)\text{.}\)
The vertical intercept is \((0,-6)\text{.}\)
The horizontal intercepts are \((2,0)\) and \((3,0)\text{.}\)
\(g(-1)=-2\text{.}\)
The solutions to \(g(x)=-6\) are the \(x\)-values where \(y=6\text{.}\) We graph the horizontal line \(y=-6\) and find the \(x\)-values where the graphs intersect. The solution set is \(\{0,5\}\text{.}\)
The solutions are all \(x\)-values where the function below (or touching) the line \(y=-6\text{.}\) The solution set is \((-\infty,0]\cup[5,\infty)\text{.}\)
The domain is \((-\infty,\infty)\) and the range is \((-\infty,0.25]\text{.}\)

Example 12.5.2 The Vertex Form of a Parabola

Recall that the vertex form of a quadratic function tells us the location of the vertex of a parabola.

State the vertex of the quadratic function \(r(x)=-8(x+1)^2+7\text{.}\)
State the vertex of the quadratic function \(u(x)=5(x-7)^2-3\text{.}\)
Write the formula for a parabola with vertex \((-5,3)\) and \(a=2\text{.}\)
Write the formula for a parabola with vertex \((1,-17)\) and \(a=-4\text{.}\)

Explanation

The vertex of the quadratic function \(r(x)=-8(x+\highlight{1})^2+\lighthigh{7}\) is \((\highlight{-1},\lighthigh{7})\text{.}\)
The vertex of the quadratic function \(u(x)=5(x-\highlight{7})^2\lighthigh{-3}\) is \((\highlight{7},\lighthigh{-3})\text{.}\)
The formula for a parabola with vertex \((\highlight{-5},\lighthigh{3})\) and \(a=2\) is \(y=2(x+\highlight{5})^2+\lighthigh{3}\text{.}\)
The formula for a parabola with vertex \((\highlight{1},\lighthigh{-17})\) and \(a=-4\) is \(y=4(x-\highlight{1})^2\lighthigh{-17}\text{.}\)

Example 12.5.3 Horizontal and Vertical Shifts

Identify the horizontal and vertical shifts compared with \(f(x)=x^2\text{.}\)

\(s(x)=(x+1)^2+7\text{.}\)
\(v(x)=(x-7)^2-3\text{.}\)

Explanation

The graph of the quadratic function \(s(x)=-8(x+1)^2+7\) is the same as the graph of \(f(x)=x^2\) shifted to the left \(1\) unit and up \(7\) units.
The graph of the quadratic function \(v(x)=5(x-7)^2-3\) is the same as the graph of \(f(x)=x^2\) shifted to the right \(7\) units and down \(3\) units.

Example 12.5.4 The Factored Form of a Parabola

Recall that the factored form of a quadratic function tells us the horizontal intercepts very quickly.

\(n(x)=13(x-1)(x+6)\text{.}\)
\(p(x)=-6(x-\frac{2}{3})(x+\frac{1}{2})\text{.}\)

Explanation

The horizontal intercepts of \(n\) are \((1,0)\) and \((-6,0)\text{.}\)
The horizontal intercepts of \(p\) are \((\frac{2}{3},0)\) and \((-\frac{1}{2},0)\text{.}\)

Subsection 12.5.2 Completing the Square

In Section 12.2 we covered how to complete the square to both solve quadratic equations in one variable and to put quadratic functions into vertex form.

Example 12.5.5 Solving Quadratic Equations by Completing the Square

Solve the equations by completing the square.

\(k^2-18k+1=0\)
\(4p^2-3p=2\)

Explanation

To complete the square in the equation \(k^2-18k+1=0\text{,}\) we first we will first move the constant term to the right side of the equation. Then we will use Fact 12.2.2 to find \(\left(\frac{b}{2}\right)^2\) to add to both sides.

\begin{align*} k^2-18k+1\amp=0\\ k^2-18k\amp=-1 \end{align*}

In our case, \(b=-18\text{,}\) so \(\left(\frac{b}{2}\right)^2=\left(\frac{-18}{2}\right)^2=81\)

\begin{align*} k^2-18k\addright{81}\amp=-1\addright{81}\\ (k-9)^2\amp=80 \end{align*}

\begin{align*} k-9\amp=-\sqrt{80}\amp\text{ or }\amp\amp k-9\amp=\sqrt{80}\\ k-9\amp=-4\sqrt{5}\amp\text{ or }\amp\amp k-9\amp=4\sqrt{5}\\ k\amp=9-4\sqrt{5}\amp\text{ or }\amp\amp k\amp=9+4\sqrt{5} \end{align*}

The solution set is \(\{9+4\sqrt{5},9-4\sqrt{5}\}\text{.}\)
To complete the square in the equation \(4p^2-3p=2\text{,}\) we first divide both sides by \(4\) since the leading coefficient is 4.

\begin{align*} \divideunder{4p^2}{4}-\divideunder{3p}{4}\amp=\divideunder{2}{4}\\ p^2-\frac{3}{4}p\amp=\frac{1}{2}\\ p^2-\frac{3}{4}p\amp=\frac{1}{2} \end{align*}

Next, we will complete the square. Since \(b=-\frac{3}{4}\text{,}\) first,

\begin{equation} \frac{b}{2}=\frac{-\frac{3}{4}}{2}=-\frac{3}{8}\label{equation-completing-square-3-4ths-over-2}\tag{12.5.1} \end{equation}

and next, squaring that, we have

\begin{equation} \left(-\frac{3}{8}\right)^2=\frac{9}{64}\text{.}\label{equation-completing-square-3-over-8}\tag{12.5.2} \end{equation}

So we will add \(\frac{9}{64}\) from Equation (12.5.2) to both sides of the equation:

\begin{align*} p^2-\frac{3}{4}p\addright{\frac{9}{64}}\amp=\frac{1}{2}\addright{\frac{9}{64}}\\ p^2-\frac{3}{4}p+\frac{9}{64}\amp=\frac{32}{64}+\frac{9}{64}\\ p^2-\frac{3}{4}p+\frac{9}{64}\amp=\frac{41}{64} \end{align*}

Here, remember that we always factor with the number found in the first step of completing the square, Equation (12.5.1).

\begin{align*} \left(p-\frac{3}{8}\right)^2\amp=\frac{41}{64} \end{align*}

\begin{align*} p-\frac{3}{8}\amp=-\frac{\sqrt{41}}{8}\amp\text{ or }\amp\amp p-\frac{3}{8}\amp=\frac{\sqrt{41}}{8}\\ p\amp=\frac{3}{8}-\frac{\sqrt{41}}{8}\amp\text{ or }\amp\amp p\amp=\frac{3}{8}+\frac{\sqrt{41}}{8}\\ p\amp=\frac{3-\sqrt{41}}{8}\amp\text{ or }\amp\amp p\amp=\frac{3+\sqrt{41}}{8} \end{align*}

The solution set is \(\left\{\frac{3-\sqrt{41}}{8}, \frac{3+\sqrt{41}}{8}\right\}\text{.}\)

Example 12.5.6 Putting Quadratic Functions in Vertex Form

Write a formula in vertex form for the function \(T\) defined by \(T(x)=4x^2+20x+24\text{.}\)

Explanation

Before we can complete the square, we will factor the \(\highlight{4}\) out of the first two terms. Don't be tempted to factor the \(4\) out of the constant term.

\begin{align*} T(x)\amp=\highlight{4}\left(x^2+5x\right)+24 \end{align*}

Now we will complete the square inside the parentheses by adding and subtracting \(\left(\frac{5}{2}\right)^2=\frac{25}{4}\text{.}\)

\begin{align*} T(x)\amp=4\left(x^2+5x\addright{\frac{25}{4}}\subtractright{\frac{25}{4}}\right)+24 \end{align*}

Notice that the constant that we subtracted is inside the parentheses, but it will not be part of our perfect square trinomial. In order to bring it outside, we need to multiply it by \(4\text{.}\) We are distributing the \(4\) to that term so we can combine it with the outside term.

\begin{align*} T(x)\amp=4\left(\highlight{\left(\unhighlight{x^2+5x+\frac{25}{4}}\right)}-\highlight{\frac{25}{4}}\right)+24\\ \amp=4\highlight{\left(\unhighlight{x^2+5x+\frac{25}{4}}\right)}-\multiplyleft{4}\frac{25}{4}+24\\ \amp=4\left(x+\frac{5}{2}\right)^2-25+24\\ \amp=4\left(x+\frac{5}{2}\right)^2-1 \end{align*}

Note that The vertex is \(\left(-\frac{5}{2},-1\right)\text{.}\)

Example 12.5.7 Graphing Quadratic Functions by Hand

Graph the function \(H\) defined by \(H(x)=-x^2-8x-15\) by determining its key features algebraically.

Explanation

To start, we'll note that this function opens downward because the leading coefficient, \(-1\text{,}\) is negative.

Now we will complete the square to find the vertex. We will factor the \(-1\) out of the first two terms, and then add and subtract \(\left(\frac{8}{2}\right)^2=4^2=\highlight{16}\) on the right side.

\begin{align*} H(x)\amp=-\left[x^2+8x\right]-15\\ \amp=-\left[x^2+8x\addright{16}\subtractright{16}\right]-15\\ \amp=-\left[\highlight{\left(\unhighlight{x^2+8x+16}\right)}-16\right]-15\\ \amp=-\highlight{\left(\unhighlight{x^2+8x+16}\right)}-\left(-1\cdot16\right)-15\\ \amp=-\left(x+4\right)^2+16-15\\ \amp=-\left(x+4\right)^2+1 \end{align*}

The vertex is \((-4,1)\) so the axis of symmetry is the line \(x=-4\text{.}\)

To find the \(y\)-intercept, we'll replace \(x\) with \(0\) or read the value of \(c\) from the function in standard form:

\begin{align*} H(\substitute{0})\amp=-(\substitute{0})^2-8(\substitute{0})-15\\ \amp=-15 \end{align*}

The \(y\)-intercept is \((0,-15)\) and we will find its symmetric point on the graph, which is \((-8,-15)\text{.}\)

Next, we'll find the horizontal intercepts. We see this function factors so we will write the factored form to get the horizontal intercepts.

\begin{align*} H(x)\amp=-x^2-8x-15\\ \amp=-\left(x^2+8x+15\right)\\ \amp=-(x+3)(x+5) \end{align*}

The \(x\)-intercepts are \((-3,0)\) and \((-5,0)\text{.}\)

Now we will plot all of the key points and draw the parabola.

a graph of the parabola y=-x^2-8x-15 opens downward and the key features are labeled; the vertex is (-4,1);the axis of symmetry is drawn at x=-4;the y-intercept is (0,-15) and its symmetric point is (-8,-15);the x-intercepts are (-3,0) and (-5,0). — Figure 12.5.8 The graph of \(y=-x^2-8x-15\text{.}\)

Subsection 12.5.3 More on Complex Solutions to Quadratic Equations

In Section 12.3 we covered the definition of a complex number, and discussed both quadratic applications and equations where complex numbers appear as solutions.

Example 12.5.9 Applications with Real or Complex Solutions

One day, Samar was bouncing a ball inside the house. The trajectory of his bounce followed the quadratic function \(H(t)=-16t^2+24t\text{,}\) where \(H(t)\) describes the height of the ball, in feet, at time \(t\) seconds after it bounced off the ground. If the ceilings in Samar's house were \(10\) feet tall, find out if the ball will hit the ceiling.

Explanation

To find out if the ball will hit the ceiling, we need to set the formula for the function equal to \(\substitute{10}\) and solve.

\begin{align*} \substitute{H(t)}\amp=-16t^2+24t\\ \substitute{10}\amp=-16t^2+24t\\ 0\amp=-16t^2+24t-10\\ \end{align*}

This is a quadratic equation where verything is divisible by \(2\text{.}\) We will divide every term by \(2\) which can simplify the process.

\begin{align*} \divideunder{0}{2}\amp=\divideunder{-16t^2}{2}+\divideunder{24t}{2}-\divideunder{10}{2}\\ 0\amp=-8t^2+12t-5\\ \end{align*}

Since the equation doesn't seem to factor easily, we will use the quadratic formula to solve it. Note that \(\substitute{a=-8}\text{,}\) \(\substitute{b=12}\text{,}\) and \(\substitute{c=-5}\text{.}\)

\begin{align*} t\amp=\frac{-b\pm\sqrt{b^2-4ac}}{2a}\\ t\amp=\frac{-(\substitute{12})\pm\sqrt{(\substitute{12})^2-4(\substitute{-8})(\substitute{-5})}}{2(\substitute{-8})}\\ t\amp=\frac{-12\pm\sqrt{-16}}{-16}\\ \end{align*}

Note that the discriminant is negative, which means that the equation has no real solutions. Just for practice, we will finish the simplification process, but we are ready to make our conclusion here.

\begin{align*} t\amp=\frac{-12\pm\sqrt{\highlight{16}\cdot\lighthigh{-1}}}{-16}\\ t\amp=\frac{-12\pm\highlight{4}\lighthigh{i}}{-16}\\ t\amp=\frac{-12}{-16}\pm\frac{\highlight{4}\lighthigh{i}}{-16}\\ t\amp=\frac{3}{4}\mp\frac{\lighthigh{i}}{4} \end{align*}

Since the solutions to the equation are complex numbers, the reality of the situation must be that the ball never does hit the ceiling. Samar's ceiling lights are safe for now.

Example 12.5.10 Solving Equations with Complex Solutions

Solve for \(x\) in \(3x^2-12x+36=0\text{.}\)

Explanation

We will use the completing-the-square method. To do so, we first need to divide both sides by the leading coefficient, \(3\text{.}\)

\begin{align*} 3x^2-12x+36\amp=0\\ 3x^2-12x\amp=-36\\ \divideunder{3x^2}{3}-\divideunder{12x}{3}\amp=\divideunder{-36}{3}\\ x^2-4x\amp=-12\\ \end{align*}

Now we can add \(\left(\frac{b}{2}\right)^2=(-2)^2=4\) to both sides to complete the square.

\begin{align*} x^2-4x\addright{4}\amp=-12\addright{4}\\ (x-2)^2\amp=-8 \end{align*}

\begin{align*} x-2\amp=-\sqrt{-8}\amp\amp\text{ or }\amp x-2\amp=\sqrt{-8}\\ x-2\amp=-\sqrt{\highlight{4}\cdot\lighthigh{-1}\cdot2}\amp\amp\text{ or }\amp x-2\amp=\sqrt{\highlight{4}\cdot\lighthigh{-1}\cdot2}\\ x-2\amp=-\highlight{2}\lighthigh{i}\sqrt{2}\amp\amp\text{ or }\amp x-2\amp=\highlight{2}\lighthigh{i}\sqrt{2}\\ x\amp=2-\highlight{2}\lighthigh{i}\sqrt{2}\amp\amp\text{ or }\amp x\amp=2+\highlight{2}\lighthigh{i}\sqrt{2} \end{align*}

The solution set is \(\{2-\highlight{2}\lighthigh{i}\sqrt{2}, 2+\highlight{2}\lighthigh{i}\sqrt{2}\}.\)

Subsection 12.5.4 Complex Number Operations

In Section 12.4 we covered the essential algebra of complex numbers.

Example 12.5.11 Adding and Subtracting Complex Numbers

Simplify the expression \((5-3i)-(1-7i)\text{.}\)

Explanation

\begin{align*} (5-3i)-(1-7i)\amp=5-3\highlight{i}-1+7\highlight{i}\\ \amp=4+4\highlight{i} \end{align*}

Example 12.5.12 Multiplying Complex Numbers

Multiply \((3+2i)(5-6i)\text{.}\)

Explanation

We will use the FOIL method to multiply the two binomials.

\begin{align*} (1+5i)(2-7i)\amp=15-18\highlight{i}+10\highlight{i}-12\highlight{i}^2\\ \amp=15-8\highlight{i}-12(-1)\\ \amp=15-8\highlight{i}+12\\ \amp=27-8\highlight{i} \end{align*}

Example 12.5.13 Dividing Complex Numbers

Simplify the expression \(\frac{3+5i}{5-6i}\text{.}\)

Explanation

To divide complex numbers, we rationalize the denominator using the conjugate \(2+4i\text{:}\)

\begin{align*} \frac{3+5i}{5-6i}\amp=\frac{(3+5i)}{(5-6i)}\multiplyright{\frac{(5+6i)}{(5+6i)}}\\ \amp=\frac{15+18\highlight{i}+25\highlight{i}+30\highlight{i}^2}{25+30\highlight{i}-30\highlight{i}-36\highlight{i}^2}\\ \amp=\frac{15+43\highlight{i}+30(-1)}{25-36(-1)}\\ \amp=\frac{15+43\highlight{i}-30}{25+36}\\ \amp=\frac{-15+43\highlight{i}}{61}\\ \amp=-\frac{15}{61}+\frac{43\highlight{i}}{61} \end{align*}

Subsection 12.5.5 Exercises

Graphs and Vertex Form

1

Use technology to make a table of values for the function \(K\) defined by \(K(x)={3x^{2}-2x-3}\text{.}\)

\(x\)	\(K(x)\)

2

Use technology to make a table of values for the function \(f\) defined by \(f(x)={-3x^{2}-8x+37}\text{.}\)

\(x\)	\(f(x)\)

3

Use technology to make a graph of \(f\) where \(f(x)=3x^2-6x-5\text{.}\)

4

Use technology to make a graph of \(f\) where \(f(x)=-3x^2-8x+3\text{.}\)

5

Let \(g(x)={-x^{2}+x+3}\text{.}\) Use technology to find the following.

The vertex is .
The \(y\)-intercept is .
The \(x\)-intercept(s) is/are .
The domain of \(g\) is .
The range of \(g\) is .
Calculate \(g(3)\text{.}\) .
Solve \(g(x)=2\text{.}\)
Solve \(g(x)>2\text{.}\)

6

Let \(h(x)={-x^{2}-4x-2}\text{.}\) Use technology to find the following.

The vertex is .
The \(y\)-intercept is .
The \(x\)-intercept(s) is/are .
The domain of \(h\) is .
The range of \(h\) is .
Calculate \(h(-1)\text{.}\) .
Solve \(h(x)=-5\text{.}\)
Solve \(h(x)\geq-5\text{.}\)

7

An object was launched from the top of a hill with an upward vertical velocity of \(110\) feet per second. The height of the object can be modeled by the function \(h(t)={-16t^{2}+110t+200}\text{,}\) where \(t\) represents the number of seconds after the launch. Assume the object landed on the ground at sea level. Find the answer using technology.

seconds after its launch, the object reached its maximum height of feet.

8

An object was launched from the top of a hill with an upward vertical velocity of \(130\) feet per second. The height of the object can be modeled by the function \(h(t)={-16t^{2}+130t+100}\text{,}\) where \(t\) represents the number of seconds after the launch. Assume the object landed on the ground at sea level. Find the answer using technology.

seconds after its launch, the object fell to the ground at sea level.

9

Find the vertex of the graph of

\begin{equation*} y=3\!\left(x-7\right)^{2}-6 \end{equation*}

10

Find the vertex of the graph of

\begin{equation*} y=6\!\left(x-3\right)^{2}-3 \end{equation*}

11

Write the vertex form for the quadratic function \(f\text{,}\) whose vertex is \((-7,-8)\) and has leading coefficient \(a=7\text{.}\)

\(\displaystyle{ f(x) =}\)

12

Write the vertex form for the quadratic function \(f\text{,}\) whose vertex is \((6,6)\) and has leading coefficient \(a=9\text{.}\)

\(\displaystyle{ f(x) =}\)

13

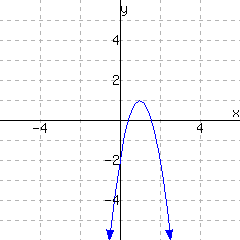
A graph of a function \(f\) is given. Use the graph to write a formula for \(f\) in vertex form. You will need to identify the vertex and also one more point on the graph to find the leading coefficient \(a\text{.}\)

eps pdf png svg tex

\(\displaystyle{ f(x) =}\)

14

eps pdf png svg tex

\(\displaystyle{ f(x) =}\)

15

Let \(h\) be defined by \(h(x)={\left(x-5\right)^{2}+5}\text{.}\)

What is the domain of \(h\text{?}\)
What is the range of \(h\text{?}\)

16

Let \(h\) be defined by \(h(x)={\left(x+2\right)^{2}-7}\text{.}\)

What is the domain of \(h\text{?}\)
What is the range of \(h\text{?}\)

17

Consider the graph of the equation \(y={\left(x-1\right)^{2}-8}\text{.}\)

Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units

left
right

and units

down
up

18

Consider the graph of the equation \(y={\left(x-3\right)^{2}+4}\text{.}\)

Compared to the graph of \(y=x^2\text{,}\) the vertex has been shifted units

left
right

and units

down
up

19

The quadratic expression \({\left(x-4\right)^{2}-4}\) is written in vertex form.

Write the expression in standard form.
Write the expression in factored form.

20

The quadratic expression \({\left(x-4\right)^{2}-1}\) is written in vertex form.

Write the expression in standard form.
Write the expression in factored form.

21

The formula for a quadratic function \(g\) is \(g(x)={\left(x-9\right)\!\left(x-3\right)}\text{.}\)

The \(y\)-intercept is .
The \(x\)-intercept(s) is/are .

22

The formula for a quadratic function \(G\) is \(G(x)={\left(x+8\right)\!\left(x+3\right)}\text{.}\)

The \(y\)-intercept is .
The \(x\)-intercept(s) is/are .

Completing the Square

23

Solve \({x^{2}-4x}={5}\) by completing the square.

24

Solve \({y^{2}+8y}={-12}\) by completing the square.

25

Solve \({y^{2}+7y+6}={0}\) by completing the square.

26

Solve \({r^{2}-5r-24}={0}\) by completing the square.

27

Solve \({12r^{2}-4r-5}={0}\) by completing the square.

28

Solve \({3t^{2}+8t+5}={0}\) by completing the square.

29

Complete the square to convert the quadratic function from standard form to vertex form, and use the result to find the function’s domain and range.

\(f(x) = {-4x^{2}+64x-248}\)

The domain of \(f\) is

The range of \(f\) is

30

Complete the square to convert the quadratic function from standard form to vertex form, and use the result to find the function’s domain and range.

\(f(x) = {-5x^{2}+100x-499}\)

The domain of \(f\) is

The range of \(f\) is

31

Graph \(f(x)=x^2-7x+12\) by algebraically determining its key features. Then state the domain and range of the function.

32

Graph \(f(x)=-x^2+4x+21\) by algebraically determining its key features. Then state the domain and range of the function.

33

Graph \(f(x)=x^2-8x+16\) by algebraically determining its key features. Then state the domain and range of the function.

34

Graph \(f(x)=x^2+6x+9\) by algebraically determining its key features. Then state the domain and range of the function.

35

Graph \(f(x)=x^2+4x+7\) by algebraically determining its key features. Then state the domain and range of the function.

36

Graph \(f(x)=x^2-2x+6\) by algebraically determining its key features. Then state the domain and range of the function.

37

Graph \(f(x)=2x^2-4x-30\) by algebraically determining its key features. Then state the domain and range of the function.

38

Graph \(f(x)=3x^2+21x+36\) by algebraically determining its key features. Then state the domain and range of the function.

39

Find the minimum value of the function

\begin{equation*} f(x)=x^{2}-7x-2 \end{equation*}

40

Find the minimum value of the function

\begin{equation*} f(x)=2x^{2}+7x+7 \end{equation*}

41

Solve the quadratic equation. Solutions could be complex numbers.

\(-10(y+8)^2 - 3 = 357\)

42

Solve the quadratic equation. Solutions could be complex numbers.

\(8(y - 3)^2 - 4 = -76\)

43

Solve the quadratic equation. Solutions could be complex numbers.

\({r^{2}-10r+32} =0\)

44

Solve the quadratic equation. Solutions could be complex numbers.

\({r^{2}-8r+19} =0\)

45

A remote control aircraft will perform a stunt by flying toward the ground and then up. Its height can be modeled by the function \(h(t)={1.7t^{2}-30.6t+133.7}\text{.}\) The plane

will
will not

hit the ground during this stunt.

46

A remote control aircraft will perform a stunt by flying toward the ground and then up. Its height can be modeled by the function \(h(t)={0.6t^{2}-10.8t+51.6}\text{.}\) The plane

will
will not

hit the ground during this stunt.

Complex Number Operations

47

Write the complex number in standard form.

\begin{equation*} (10-2i)-(5-4i) \end{equation*}

48

Write the complex number in standard form.

\begin{equation*} (-9-9i)-(-7-10i) \end{equation*}

49

Write the complex number in standard form.

\begin{equation*} (-6+4i)(2+6i) \end{equation*}

50

Write the complex number in standard form.

\begin{equation*} (-4-3i)(10) \end{equation*}

51

Rewrite the following expression into the form of a+b\(i\text{:}\)

\(\displaystyle{ \frac{{-1-8i}}{{-2-5i}} = }\)

52

Rewrite the following expression into the form of a+b\(i\text{:}\)

\(\displaystyle{ \frac{{3+6i}}{{7-5i}} = }\)